>	restart:#"m13_p05"

Calcular el calor transmitido entre dos superficies opacas semirreflectantes cuando se interponen n (n = 0,1,2,...) escudos radiativos, en los casos siguientes:

a) Geometría plana y reflectancia nula de todas las placas (incluyendo las paredes).

b) Geometría cilíndrica o esférica y reflectancia nula.

c) Geometría plana y paredes grises.

Find the effect on the radiative heat transfer between two surfaces when interposing several shields, in the following cases:

a) Infinite planar geometry, considering all surfaces as blackbodies.

b) Spheres or infinite cylinders geometry, considering all surfaces as blackbodies.

c) Infinite planar geometry, in the general case of grey surfaces.

Datos:

>	read`../therm_eq.m`:read`../therm_const.m`:read`../therm_proc.m`:with(therm_proc):

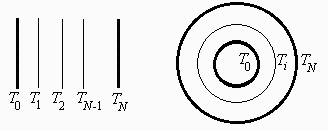
Fig. 1. Notation and geometry (planar, cylindrical).

a) Infinite planar geometry, considering all surfaces as blackbodies.

Let use the notation of Fig. 1, with T0 and TN fixed, and the number of intermediate radiation shields being N-1. We assume steady state, with negligible thermal resistance of the shield material itself, and no other heat source and sinks that the end plates. In absence of shields (N=1), the heat flow per unit area is q=Q/A=sigma·(T0^4-TN^4). In the case of shields, and since the same heat flux must be constant along the path, we have

>	eq1:=q[0]=sigma*(T[0]^4-T[N]^4);eq||i:=q[N-1]=sigma*(T[i]^4-T[i-1]^4);eqN:=q[N-1]=Sum(rhs(eq||i),i=1..n+1);eqN:=q[N-1]=sigma*(T[0]^4-T[N]^4)/N;

	(1)

where the last equality is obtained by summing up all numerators and all denominators. The conclusions are that heat transfer is reduced, being the undisturbed value divided by the number of shields, which attain the series of temperatures:

>	eqq:=q[N-1]=q0/N;eqq_:=rhs(eqN)=sigma*(T[0]^4-T[i]^4)/i;T[i]:=(((N-i)*T[0]^4+i*T[N]^4)/N)^(1/4);T[i]:='T[i]':

	(2)

where the intermediate result was obtained by summation of numerators and denominators from 1 to i instead of from 1 to N as before.

For instance, if T0=700 K and TN =300 K, we get T1=594 K in case of only one shield, T1=635 K and T2=541 K in case of two shields, and so on.

b) Spheres or infinite cylinders geometry, considering all surfaces as blackbodies.

In this case, surface areas enter into the analysis. With the same assumptions as before, the heat flow in absence of shields (N=1) is . Notice that the heat transfer only depends on the smaller area A0 and not on AN. In the case of shields, and since the same heat flow (but not per unit area) must be constant along the path, we have:

>	eq1:=Q[0]=A[0]*sigma*(T[0]^4-T[N]^4);eq||i:=Q[N-1]=A[i]*sigma*(T[i]^4-T[i-1]^4);eqN:=Q[N-1]=Sum(rhs(eq||i),i=1..n+1);eqN:=Q[N-1]=sigma*(T[0]^4-T[N]^4)/Sum(1/A[i],i=1..N-1);

	(3)

The generic solution is:

>	eqQ:=Q[N-1]=Q0/Sum(A[0]/A[i],i=1..N-1);T[i]:=(((Sum(A[0]/A[i],i=1..N-1)-Sum(A[0]/A[i],i=1..'i-1'))*T[0]^4+Sum(A[0]/A[i],i=1..'i-1')*T[N]^4)/Sum(A[0]/A[i],i=1..N-1))^(1/4);T[i]:='T[i]':

	(4)

which, for the case of only one shield (N=2) of area A1=alpha*A0, reduces to:

>	eqA:=alpha=A[1]/A[0];eqQ:=Q[1]=Q0/(1+1/alpha);T[i]:=((T[0]^4/alpha+T[2]^4)/(1+1/alpha))^(1/4);

	(5)

Notice that the shield is most effective when close to the smaller area (alpha→1), where the heat transfer reduces to a half ( ); on the contrary, when close to the larger surface, the shield has no effect. For instance, for spheres with T0=700 K, A0=1 m2, TN=300 K, AN=3 m2, and alpha=2 (A1=2 m2), we get Q0=13 kW and T1=541 K.

c) Infinite planar geometry, in the general case of grey surfaces.

Let each surface  has emissivities epsilonminus (left side) and epsilon_plus (right face).

>	eq||i:=q[N-1]=(T[i]^4-T[i-1]^4)/((1/sigma)*(1/epsilon[i,m]+1/epsilon[i-1,p]-1));eqSum:=q[N-1]=Sum(rhs(eq||i),i=1..N);q[N-1]:=(T[N]^4-T[0]^4)/((1/sigma)*Sum((1/epsilon[i,m]+1/epsilon[i-1,p]-1),i=1..N));q[1]:=value(subs(N=2,q[N-1]));

	(6)

where the last equation gives the heat flux when only 1 shield is in between.

>