>	restart:#"m13_p38"

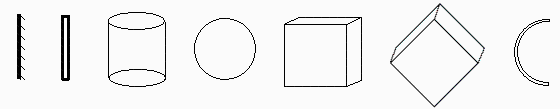
Find the steady temperature at 1 AU, for an isothermal blackbody with the following geometries: planar one-side surface (i.e. rear insulated), plate, cylinder, sphere, and cubic box in its three symmetric orientations

Datos:

>	read`../therm_eq.m`:read`../therm_const.m`:read`../therm_proc.m`:with(therm_proc):

>	dat:=[E=1370*W_/m_^2];

>	dat:=op(dat),Const,SI2,SI1:

a) Find the steady temperature at 1 AU, for an isothermal blackbody with the following geometries:

Energy balance, and steady state temperature for a blackbody:

>	eqEB:=alpha*Afrontal*E=epsilon*Aext*sigma*Tst^4;Tst:=(Afrontal*E/(Aext*sigma))^(1/4);

Planar one side.

For one-side planar surface of area A with its normal tilted an angle beta to Sun rays, frontal area Af=Acos, and emitting area Ae=A, thence

>	Afrontal:=A*cos(beta);Aext:=A;Tst_:=Tst;Tst0_:=evalf(subs(beta=0,dat,SI0,%))*K_;'Tst0_'=TKC(%);

Planar two sides.

For a plate emitting from both sides,

>	Afrontal:=A*cos(beta);Aext:=2*A;Tst_:=Tst;Tst0_:=evalf(subs(beta=0,dat,SI0,%))*K_;'Tst0_'=TKC(%);

Cylinder.

For a cylinder of diameter D and length L with its axis tilted an angle beta to Sun rays, with all its surfaces emitting.

>	Afrontal:=(Pi*D^2/4)*cos(beta)+Pi*D*L*sin(beta)/2;Aext:=2*(Pi*D^2/4)+Pi*D*L;Tst_:=Tst;Tst0_DL:=evalf(subs(beta=0,L=D,dat,SI0,%))*K_;'Tst0_'=TKC(%);Tst90_DL:=evalf(subs(beta=Pi/2,L=D,dat,SI0,Tst_))*K_;'Tst90_'=TKC(%);

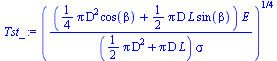Sphere.

>	Afrontal:=Pi*D^2/4;Aext:=Pi*D^2;Tst_:=Tst;Tst0_:=evalf(subs(beta=0,dat,SI0,%))*K_;'Tst0_'=TKC(%);

Cube. Frontal.

For a frontal cube, i.e. an hexahedron of face area A, with all its surfaces emitting, Af=A and Ae=6A, thence:

>	Afrontal:=A;Aext:=6*A;Tst_:=Tst;Tst0_:=evalf(subs(beta=0,dat,SI0,%))*K_;'Tst0_'=TKC(%);

Cube at 45º.

For a cube tilted 45º, i.e. an hexahedron with two opposite edges and the Sun in the same plane, Af=sqrt(2)*A, and Ae=6A, thence:

>	Afrontal:=A*sqrt(2);Aext:=6*A;Tst_:=Tst;Tst0_:=evalf(subs(beta=0,dat,SI0,%))*K_;'Tst0_'=TKC(%);

Cube point-to-point.

For a cube pointing to the Sun, i.e. an hexahedron with two opposite vertices and the Sun in the same straight line (3 lit faces tilted 54.7º, instead of 2 lit faces tilted 45º in the previous case),Af=sqrt(3)*A  and Ae=6A, thence:

>	Afrontal:=A*sqrt(3);Aext:=6*A;Tst_:=Tst;Tst0_:=evalf(subs(beta=0,dat,SI0,%))*K_;'Tst0_'=TKC(%);

Notice that only fully-convex surfaces have been considered; otherwise, view factors enter into play. For instance, consider a hemispherical shell with its symmetry axis aligned with the Sun.

Afrontal:=Pi*D^2/4;Aext:=Pi*D^2;eqEB:=alpha*Afrontal*E=epsilon*(F[1,ext]*A[1,ext]+F[1,int]*A[1,int])*sigma*Tst^4;F[1,ext]:=1;F[1,int]:=1/2;A[1,ext]:=Pi*D^2/2;A[1,int]:=Pi*D^2/2;Tst:=(Afrontal*E/((F[1,ext]*A[1,ext]+F[1,int]*A[1,int])*sigma))^(1/4);Tst_:=evalf(subs(dat,SI0,%))*K_;'Tst_'=TKC(%);

Notice that a hollow hemisphere gets warmer than a spherical shell, having the same frontal area and exposed area, at 26 ºC instead of at 6 ºC, because the concave part re-radiates to itself.

>